
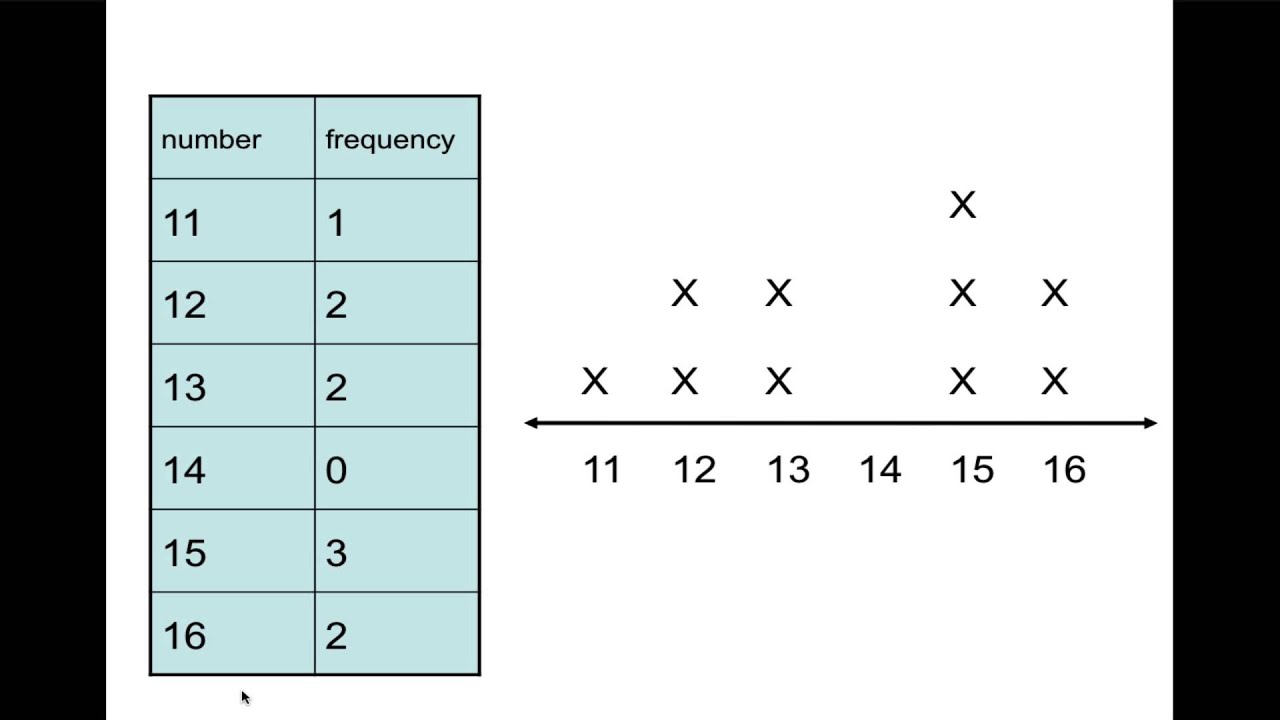
There is not a set procedure for determining the number of bars or bar width/bin size however, consistency is key when determining which data values to place inside each interval. The width of each bar is also referred to as the bin size, which may be calculated by dividing the range of the data values by the desired number of bins (or bars). Many histograms consist of five to 15 bars or classes for clarity. To construct a histogram, first decide how many bars or intervals, also called classes, represent the data. Ninety to 100 percent is a quantitative measures. Thus, 7.5 percent of the students received 90 to 100 percent. Ahab's English class of 40 students received from ninety to 100 percent, then, , and. = total number of data values (or the sum of the individual frequencies), andįor example, if three students in Mr.Remember, frequency is defined as the number of times an answer occurs. The relative frequency is equal to the frequency for an observed value of the data divided by the total number of data values in the sample. In a skewed distribution, the mean is pulled toward the tail of the distribution. The shape of the data refers to the shape of the distribution, whether normal, approximately normal, or skewed in some direction, whereas the center is thought of as the middle of a data set, and the spread indicates how far the values are dispersed about the center. The histogram (like the stemplot) can give you the shape of the data, the center, and the spread of the data. The graph will have the same shape with either label.
The vertical axis is labeled either frequency or relative frequency (or percent frequency or probability). The horizontal axis is more or less a number line, labeled with what the data represents, for example, distance from your home to school. It has both a horizontal axis and a vertical axis. One advantage of a histogram is that it can readily display large data sets.Ī histogram consists of contiguous (adjoining) boxes.
Find outliers on a stem plot series#
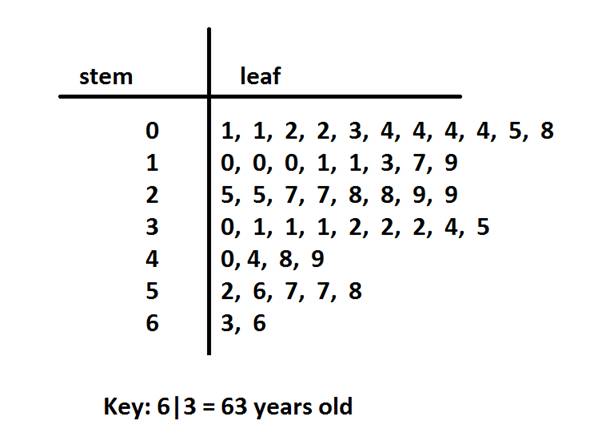
Histograms, Frequency Polygons, and Time Series Graphsįor most of the work you do in this book, you will use a histogram to display the data. The frequency of data values for each stem provides information about the shape of the distribution.įor Susan Dean's spring precalculus class, scores for the first exam were as follows (smallest to largest):ģ3, 42, 49, 49, 53, 55, 55, 61, 63, 67, 68, 68, 69, 69, 72, 73, 74, 78, 80, 83, 88, 88, 88, 90, 92, 94, 94, 94, 94, 96, 100 Make sure the leaves show a space between values, so that the exact data values may be easily determined. Then write the leaves in increasing order next to their corresponding stem. Draw a vertical line to the right of the stems. Write the stems in a vertical line from smallest to largest. The decimal 9.3 has stem nine and leaf three. Likewise, the number 5,432 has stem 543 and leaf two. For example, 23 has stem two and leaf three. The stem consists of the leading digit(s), while the leaf consists of a final significant digit. To create the plot, divide each observation of data into a stem and a leaf. It is a good choice when the data sets are small. One simple graph, the stem-and-leaf graph or stemplot, comes from the field of exploratory data analysis. Stem-and-Leaf Graphs (Stemplots), Line Graphs, and Bar Graphs


 0 kommentar(er)
0 kommentar(er)
